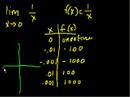
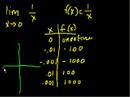
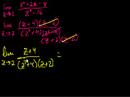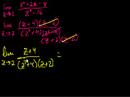Lec 12 - Epsilon Delta Limit Definition 2
Epsilon Delta Limit Definition 2 Using the epsilon delta definition to prove a limit
Video is embedded from external source so embedding is not available.
Video is embedded from external source so download is not available.
Channels: Mathematics
Tags: Epsilon Delta Limit Definition 2
Uploaded by: khancalculus ( Send Message ) on 05-09-2012.
Duration: 10m 56s
Here is the next lecture for this course
Lec 11 - Epsilon Delta Limit Definition 1
12:48 | 4646 viewsChemical Science - Covalent Bonds - Lectu ...
47:12 | 16567 viewsExploring the Sun in High Definition
03:06 | 6000 viewsLec 4 - Limit Examples (part 1)
08:56 | 3368 viewsLec 5 - Limit Examples (part 2)
06:57 | 3872 viewsLec 7 - Limit Examples w/ brain malfuncti ...
15:02 | 3955 viewsLec 3 - Limit Examples (part 1)
08:56 | 3141 viewsLec 4 - Limit Examples (part 2)
06:57 | 2921 viewsLec 5 - Limit Examples (part3)
09:58 | 2711 viewsLec 6 - Limit Examples w/ brain malfuncti ...
15:02 | 3394 viewsLec 6 - The unit circle definition of tri ...
09:07 | 4628 viewsLec 7 - Unit Circle Definition of Trig Fu ...
10:11 | 4503 viewsLec 41 - Dirac Delta Function
17:48 | 3476 viewsLec 42 - Laplace Transform of the Dirac D ...
12:13 | 3219 viewsLec 25 - Central Limit Theorem
09:49 | 3210 viewsNo content is added to this lecture.
This video is a part of a lecture series from of khan
Lecture list for this course
Lec 1 - Newton Leibniz and Usain Bolt
Lec 2 - Introduction to Limits (HD)
Lec 3 - Introduction to Limits
Lec 4 - Limit Examples (part 1)
Lec 5 - Limit Examples (part 2)
Lec 6 - Limit Examples (part3)
Lec 7 - Limit Examples w/ brain malfunction on first prob (part 4)
Lec 11 - Epsilon Delta Limit Definition 1
Lec 13 - Calculus: Derivatives 1 (new HD version)
Lec 14 - Calculus: Derivatives 2 (new HD version)
Lec 15 - Calculus: Derivatives 2.5 (new HD version)
Lec 16 - Derivative Intuition Module
Lec 17 - Calculus: Derivatives 1
Lec 18 - Calculus: Derivatives 2
Lec 19 - Calculus: Derivatives 3
Lec 28 - Proof: d/dx(ln x) = 1/x
Lec 29 - Proof: d/dx(e^x) = e^x
Lec 30 - Proofs of Derivatives of Ln(x) and e^x
Lec 31 - Extreme Derivative Word Problem (advanced)
Lec 32 - Implicit Differentiation
Lec 33 - Implicit Differentiation (part 2)
Lec 34 - More implicit differentiation
Lec 35 - More chain rule and implicit differentiation intuition
Lec 36 - Trig Implicit Differentiation Example
Lec 37 - Calculus: Derivative of x^(x^x)
Lec 38 - Introduction to L'Hopital's Rule
Lec 39 - L'Hopital's Rule Example 1
Lec 40 - L'Hopital's Rule Example 2
Lec 41 - L'Hopital's Rule Example 3
Lec 42 - Maxima Minima Slope Intuition
Lec 43 - Inflection Points and Concavity Intuition
Lec 45 - Calculus: Maximum and minimum values on an interval
Lec 46 - Calculus: Graphing Using Derivatives
Lec 47 - Calculus Graphing with Derivatives Example
Lec 48 - Graphing with Calculus
Lec 49 - Optimization with Calculus 1
Lec 50 - Optimization with Calculus 2
Lec 51 - Optimization with Calculus 3
Lec 52 - Optimization Example 4
Lec 53 - Introduction to rate-of-change problems
Lec 54 - Equation of a tangent line
Lec 55 - Rates-of-change (part 2)
Lec 56 - Ladder rate-of-change problem
Lec 58 - The Indefinite Integral or Anti-derivative
Lec 59 - Indefinite integrals (part II)
Lec 60 - Indefinite Integration (part III)
Lec 61 - Indefinite Integration (part IV)
Lec 62 - Indefinite Integration (part V)
Lec 63 - Integration by Parts (part 6 of Indefinite Integration)
Lec 64 - Indefinite Integration (part 7)
Lec 65 - Another u-subsitution example
Lec 66 - Introduction to definite integrals
Lec 67 - Definite integrals (part II)
Lec 68 - Definite Integrals (area under a curve) (part III)
Lec 69 - Definite Integrals (part 4)
Lec 70 - Definite Integrals (part 5)
Lec 71 - Definite integral with substitution
Lec 72 - Integrals: Trig Substitution 1
Lec 73 - Integrals: Trig Substitution 2
Lec 74 - Integrals: Trig Substitution 3 (long problem)
Lec 75 - Periodic Definite Integral
Lec 76 - Simple Differential Equations
Lec 77 - Solid of Revolution (part 1)
Lec 78 - Solid of Revolution (part 2)
Lec 79 - Solid of Revolution (part 3)
Lec 80 - Solid of Revolution (part 4)
Lec 81 - Solid of Revolution (part 5)
Lec 82 - Solid of Revolution (part 6)
Lec 83 - Solid of Revolution (part 7)
Lec 84 - Solid of Revolution (part 8)
Lec 85 - Sequences and Series (part 1)
Lec 86 - Sequences and series (part 2)
Lec 87 - Maclauren and Taylor Series Intuition
Lec 88 - Cosine Taylor Series at 0 (Maclaurin)
Lec 89 - Sine Taylor Series at 0 (Maclaurin)
Lec 90 - Taylor Series at 0 (Maclaurin) for e to the x
Lec 91 - Euler's Formula and Euler's Identity
Lec 92 - Visualizing Taylor Series Approximations
Lec 93 - Generalized Taylor Series Approximation
Lec 94 - Visualizing Taylor Series for e^x
Lec 95 - Polynomial approximation of functions (part 1)
Lec 96 - Polynomial approximation of functions (part 2)
Lec 97 - Approximating functions with polynomials (part 3)
Lec 98 - Polynomial approximation of functions (part 4)
Lec 99 - Polynomial approximations of functions (part 5)