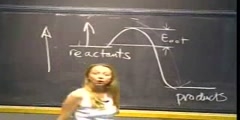Chemical Science - Breakdown of Octet Rule - Lecture 13
Principles of Chemical Science/n * Email this page/nVideo Lectures - Lecture 13/nTopics covered: /nBreakdown of Octet Rule/nInstructor: /nProf. Sylvia Ceyer/nTranscript - Lecture 13/nThe goal is to count up all of the valence electrons that you have in a molecule and then distribute those valence electrons so that between each two atoms there are at least two electrons./nAnd then what you do is you take the rest of the electrons and distribute them around the molecule so that each atom has an inner gas configuration around it. That's usually an octet, unless you're dealing with hydrogen. That's the main idea. And so what that means is that you have to know what those valence electrons are./nAnd so that is something that you should get used to knowing so you could just really write that down immediately. But we also saw last time that a very critical concept was this concept here of formal charge. Formal charge is a measure of how much an atom has gained or lost an electron when it has formed a bond in a particular structure./nAnd we saw how this idea of formal charge was really critical in being able to determine what the lowest energy structure was. In fact, what was the lowest energy skeletal structure? Often times, when you start out, you don't know what atoms are connected to each other. You have to take a guess. You take a guess. You put out the Lewis structure./nYou draw out the Lewis structure and then you calculate the formal charge. And if you find on that Lewis structure that any atom has a formal charge, a minus two or a plus two or minus three or plus three, that cannot possibly be the lowest energy structure. And then you start again. You find another skeletal structure, draw out the Lewis diagram, calculate the formal charges./nAnd you keep doing that until you find a skeletal structure that gives you the lowest set or the lowest absolute values of formal charges. This is a very important concept. That's how you do it. Now, we also saw last time that we were able to draw a couple of different skeletal structures were the set of formal charges was the same./nExcept that in those two different Lewis structures, one structure had a formal negative charge on an oxygen and the other one had it on the nitrogen. In that case, we want to always make sure we put the negative formal charge on the most electronegative element. And then, finally, in our discussion of formal charge, we were talking about this ion here, NO3-./nAnd this formal charge concept helped us to understand when we had a resonance structure. For example, we did a Lewis structure that looked like this. And we put this double bond here between one nitrogen and one of the oxygens, but we realized we could have also put it between this nitrogen and oxygen and we realized we could have put it between this oxygen and this nitrogen./nAnd, of course, in all three cases we've got the same set of formal charges. They look identical. So what happens in this case? Well, when you've got this situation where it looks like you've got three different structures and the formal charges are the same, well, this is a hint that you have a resonance structure. That is that these two electrons here, these two extra electrons are not sitting between just one nitrogen and an oxygen./nBut rather these two electrons are delocalized over all three bonds. That is to say these three bonds, nitrogen-oxygen, nitrogen-oxygen, nitrogen-oxygen are all equivalent. It is not the case where one of the bonds is a double bond and the other two are single bonds. Rather, those extra two electrons are distributed equally over all three bonds./nAnd so each one of these bonds kind of looks like a bond and a third. It's a little bit shorter than a single bond and a little bit stronger than a single bond, but not as short as a double bond and not as strong as a double bond. When you have this situation of three sets, three different structures, three different Lewis structures but same set of absolute values, that's a clue you've got a resonance structure./nOK? Yes?/nNo, the lone pairs here are not. They actually reside, they're most close to the actual atom that they're shown. It's only the bonding electrons that exhibit this delocalization./nAll right. Now what we want to do today is I want to show you some exceptions to the octet rule. There are always exceptions. And we started out saying that these Lewis diagrams and the octet rule, that worked 90% of the time. Now I want to show you the other 10% when it actually doesn't work. And so here is the first exception when it doesn't work./nIt doesn't work when you have an odd number of valence electrons because these Lewis diagrams are based on the idea that a bond shares, a bond is composed of two electrons, and those two electrons are shared between the two atoms. And so if you've got an odd number of valence electrons this whole idea breaks down. For example, the methyl radical, CH3 dot./nA methyl radical has an odd number of valence electrons. It has seven valence electrons. It is because it has that one unpaired electron that it's actually really very reactive. And so if you try to use your Lewis rules that we had on this kind of situation where you've got an odd number of valence electrons, everything really just breaks down./nSo if you're given a structure to draw and you've got an odd number of valence electrons forget it. You really cannot use the octet rule and then the Lewis diagrams to do that. You need a more sophisticated scheme for looking at the bonding of molecules which have an odd number of valence electrons. And we're going to look at some of those more sophisticated schemes in a couple of days or so. So that's one exception./nBut here comes a second exception, and this is an exception to the octet rule. The octet rule is going to break down, but we are still going to draw a Lewis diagram. And I will show you how. The octet rule is going to break down for these elements in group 13. The octet rule is going to break down for boron, aluminum, gallium, indium in the group 13./nYou come to a problem where you've got to draw a Lewis diagram for a group 13 element. Do you know what should happen in your mind? Buzzers ought to go off, buzz, buzz, buzz meaning there's going to be an exception here. I mean this. Really, if you see boron, aluminum, you're on heightened alert here. Something unusual could happen. Let me explain this. Suppose we are writing the Lewis structure for BF3, we need the skeletal structure first. Well, I said last time that the fluorines, they're just almost always terminal atoms. Good guess. Put the boron in the center. Now let's count up the number of valence electrons./nWell, we have four atoms here. Each of the three fluorines are bringing in seven valence electrons and the boron is bringing in three. We've got a total number of 24 valence electrons here. I just drew them out. Now we want to count up our step three here, the number of electrons that we would have to have if each one of these atoms had an octet around it. So we've got four atoms. Each one wants eight./nSo we would have to have 32 electrons. To calculate the number of bonding electrons in step four we subtract the number of electrons that we'd have to have for an inner gas configuration from the number of valence electrons that we do have. And that is eight. And what I did last time is I put a square here around the valence electrons that are going to be bonding electrons./nAnd our next step in the Lewis diagram said we're going to assign those bonding electrons, two to each bond. Let's do that. Well, we just did it. Here is two, here is two, here is two. We used up six of the bonding electrons. And our next step says do we have any remaining bonding electrons. Well, yeah, we do. We've got two bonding electrons here that we haven't used./nAnd we said last time in this case what we usually do, or what we do is that we assign those electrons so that we have multiple bonds. We have a double bond. For example, we're going to choose one of these boron-fluorine bonds and put in another two electrons so that we have a double bond between the boron and the fluorine. That's what our rules said last time, so let's follow them for a moment. So we did that. Now we've used up all of our bonding electrons./nDo we have any valence electrons left? We sure do. Gobs of them. We only used eight of them for the bond so we've got 16 valence electrons. That means we're going to have to make eight lone pairs. And we distribute them around each atom in the molecule so that each atom has an octet. So let's do that. Here we've got eight around fluorine, eight around this fluorine, there's eight around the boron and there is eight around the fluorine./nEverything looks normal. Everything looks great. Now let's calculate the formal charge. That is something else we've got to check. Formal charge is number of valence electrons minus the number of electrons that are lone pairs minus the number of bonding electrons divided by two. Let's calculate that for boron in this structure./nSo we said boron has three valence electrons. How many lone pairs does boron have in this structure? How many little pairs? None. They're all bonding electrons. How many bonding electrons does boron have? Eight. Eight divided by two is four. The formal charge on this boron is minus one, so we're going to put a minus one right there./nAnd then if you proceed to calculate the formal charge on this doubly bonded fluorine right here, you would find that that formal charge was plus one. And if you go and you calculate the formal charges on the singly bonded fluorines, you would find that that is zero. So I will put a zero and a zero there. And now, remember, last time we said that the sum of those formal charges has to equal the overall charge on the molecule./nIf it doesn't you've done something wrong somewhere. And so I just summed them up. That sum is zero. The overall charge on BF3 is zero. We did things correctly. But now when you look at this you say, well, this looks like NO3- in the sense that I could have taken these two electrons and put them in this bond to make that structure. If I did, I'd have the same set of formal charges./nOr I could have taken those two electrons and put them right here like that. If I did so, I would still have the same set of formal charges. Look, this looks like one of these resonance structures that I just told you about. That's what it looks like. Why not resonance? Well, why not? Because this beeper is going off in your head, beep, beep, beep, this is boron./nBoron is an exception. Let me show you how it is an exception. Experimentally, if you look at these boron-fluorine bonds, you would not see that there would be one double bond and two single bonds. You would not find a boron-fluorine bond that looks like a double bond and two that look like single bonds./nYou would also not find that these three bonds were a bond and a third like in NO3-. Experimentally, you would find that these boron-fluorine bonds all have the same length but they are typical of a single bond. Unlike in NL3- where it was a bond and a third, these boron-fluorine bonds all look like single bonds in terms of their lengths, in terms of their strengths./nVery different than NL3-. Given that experimental observation, what are we going to do? Well, we're going to write a structure here where we're going to give every atom in that structure an octet except for the boron. So what did I do?/nI took the extra two electrons here, removed it from the boron-fluorine bond and gave it just to the fluorine so that every fluorine or every atom in this molecule has an octet, except for this outlier boron. Still got the same number of electrons, everything is fine, but I moved these electrons from here to the fluorine./nLet me calculate the formal charge here. If I do that, I find that the formal charge on boron is zero now instead of minus one. I find that the formal charge on this fluorine is zero, this fluorine is zero, and this fluorine, hey, that's zero. I've got a structure with all zero formal charges. That is awesome. [LAUGHTER]/nThe octet rule just broke down here. And that is the case for the elements in group 13, or that is often times the case for the elements in group 13. In BS4-, for example, the octet rule doesn't break down. But in BF3, BCL3, aluminum trichloride same thing./nThe group 13 elements are octet deficient. So that's an exception that you have to know about. And, in fact, this is the structure. Experimentally we can tell that. And it's the lowest energy structure. We've got a set of zero formal charges. So that's group 13. That is our second exception./nThis just repeats that. Great. Now here is our third exception. So we've got an exception now where we don't put an octet around some atoms. And now this is the opposite case. The third exception is where we're going to put more than an octet around an atom. We're going to call this valence shell expansion./nAnd the example we're going to use is phosphorus pentachloride. Let's draw the Lewis structure first, and then we will see how our rules are going to kind of break down. For phosphorus pentachloride, let's put the phosphorous in the center because the chlorines are usually terminal. Not always but usually. So let's start there./nWe do that. Let's calculate the number of valence electrons. Well, fluorine, it brings in seven valence electrons. There are five of them. Phosphorus, it brings five valence electrons into the party. We've got 40 valence electrons. How many electrons would we have to have if we had an inner gas configuration around each atom? Well, we've got six atoms. Six times eight is 48./nSo what do our rules say about the number of bonding electrons? Well, our rules say that we've got eight bonding electrons because 48 minus 40 is eight. Well, that's great. Eight bonding electrons, we can make four bonds. But we've got a problem. You can see already that we're going to have to make at least five bonds so we've got a problem. Our kind of formula here for writing these Lewis structures broke down./nIt's clear we're going to have to make ten bonds, but in our procedure here it let us make only four bonds. So what are we going to do? Fudge. Good. That's exactly what we're going to do. We're going to fudge. We're going to forget our rules. We're going to draw five bonds, each one to chlorine. So now, of our 40 valence electrons, ten of them have been used as bonding electrons./nThat means we've got 30 valence electrons leftover. We're going to have to distribute them around the molecule so that each atom, except the phosphorus, has an octet around it. We will do that. Here are six for that chlorine. Now we used another six, 12 for that chlorine./nAnother six for that chlorine, 18. Another six for that chlorine, 24. Another six for that chlorine, 30. We just used them all up. Let's calculate the formal charges. The formal charge on phosphorus is number of valence electrons on phosphorus, which is zero. Number of lone pairs on phosphorus, which is zero. And number of bonding electrons divided by two on phosphorus. Well, there are ten bonding electrons./nTwo, four, six, eight, ten divided by two, that's five. Formal charge on phosphorus is zero. And now if you go and you calculate the formal charges on the chlorines, they are all zero. And we've got another awesome looking structure here, a set of zero formal charges for phosphorus pentachloride. That is very nice except for the fact that we've got more than an octet around the phosphorus./nThe phosphorus has what we call an expanded shell. And that is the case, that will be often the case for elements which have their outer most electrons in a shell where the principle quantum number is three or greater. Those elements have empty d states./nEmpty d states that can accommodate more than eight electrons that can accommodate more than an octet. And phosphorus is one of those elements. So when you start to get to the larger elements like phosphorus, like the next example iodine that I am going to show you, we can put more than an octet around those larger elements. Let's do another example here with this valance shell expansion./nHere is the IF4- ion. How do you draw, first of all, a skeletal structure? What is a good guess for a skeletal structure here? Well, chlorines are always terminal. Let's put them on the outside and the iodine in the middle./nIs there a question over there? Can I help you out? Any questions right now? OK. Let's try it. Let's count up the number of valence electrons. Well, we've got one, two, three, four, five atoms. And they are halogens. Halogens bring in seven valence electrons. Five times seven is 35. But, and this is important, we've got an extra charge here./nThis is an ion. It is minus one. And so we're going to have to add one to the number of valence electrons. We've got 36 valence electrons. Now, the number of electrons of each had an octet so that would be 40 because we have five. Five times eight is 40. According to our rules then, the number of bonding electrons is 40 minus 36 which is six./nWhich is four. [LAUGHTER] It's four. But what do we need? We need eight bonding electrons because we've got to make four bonds. Here again we have a breakdown in our rules. Something is wrong. We are going to have to fudge, as somebody said. We're going to ignore our rules. We've got to make eight bonds. Oh, jeez./nWe've got to make four bonds so we need eight electrons. Let's make them. There they are. So we used eight of the valence electrons as bonding electrons. 36 minus eight is 28. We've got 28 remaining valence electrons or 14 lone pairs. We're going to distribute them around that fluorine. There are six, that fluorine, there is another six, and that's 12. That fluorine is 18. This fluorine is 24./nWe just used up 24 of those valence electrons, but we haven't used them all. We've got four valence electrons remaining. Where are they going to go? Well, where we are going to put those four remaining valence electrons are around the iodine right there. We're going to give them to the iodine, and the iodine is going to have two lone pairs./nSo the iodine here has four more electrons around it. And we can do that again because the iodine has got some empty five d states that can accept these electrons, and it does. Everything has got an octet here except for the iodine. Let's check the formal charges and make sure we did everything correctly./nCalculate the formal charge on iodine. Well, number of valence electrons, seven. Minus the number of lone pairs. Look at how many lone pair electrons we have on the iodine, two, four. We've got four lone pair electrons on iodine. Minus one-half the number of bonding electrons on iodine. Well, we've got eight bonding electrons. Here they are, two, four, six, eight. These red ones don't count. They are the lone pairs./nEight over two is four. The formal charge on iodine is minus one. If you go and you calculate the formal charges on fluorine, they are all zero. Some of those are minus one. That is indeed the overall charge on this iodine tetrafluoride. So we did it right./nHere is another case where there is an octet around each one of the atoms, except for that larger atom, the iodine, which has actually two, four, six, eight, twelve electrons around it. Yes?/nNo, these aren't d electrons necessarily. These are in empty states here. There is plenty of room even to put some more in the case of iodine. We saw that these Lewis diagrams, the octet idea works 90% of the time, but three exceptions. An odd number of electrons?/nForget it. You cannot use it. We're going to find some more sophisticated models for bonding. It doesn't work on group 13. Group 13 elements often have less than an octet. And then, finally, if you get to the heavier elements like iodine, like phosphorus that we looked at, like chromium is one example in the notes that I didn't work through./nBut you will work through that in recitation. You've got some empty d states. You can put more than an octet around those heavier atoms. So that is what I wanted to say here about the Lewis diagrams. And next, on Friday we're going to start looking at a little bit more sophisticated model for chemical bonding, which is molecular orbital theory./nBut right now what I want to do is I want to talk about one other kind of classical model for chemical bonds. It is a model that works only for a very ionic bond like sodium chloride. That is what we're going to talk about here./nAnd, in particular, it's a model that is simple but accurate in terms of giving us a physical picture of actually how this chemical bond forms. Let's talk about it. We're going to talk about the formation of a bond between a sodium atom and a chlorine atom. How does this happen? Well, we've got sodium and chlorine coming together./nWhat happens here is this sodium ejects an electron and its spheres the chlorine, and that electron now is stuck on the chlorine. And so now the chlorine is Cl-. It's pretty big. Sodium is Na+. It's small. And once that happens that sodium just ropes that chlorine right in. It just pulls it right into it./nIt is called the harpoon mechanism. Now, once that electron jump happens of course these two ions come together. What is this rope in between the sodium plus and the chlorine minus? Well, that rope is the Coulomb interaction, right? Because once that electron has jumped from sodium to the chlorine, we have a positive charge and a negative charge./nAnd they are attracted. And they just zoom right in together until you get to the bond length and they form a chemical bond. That is literally what happens. The sodium ejects an electron, that jumps to the chlorine, and then the two just zoop right into each other and form a chemical bond called the harpoon mechanism./nThis is a mechanism here that was really shown experimentally by Dudley Herschbach here at Harvard in the Chemistry Department, John Polanyi in Toronto and Yuan Lee in UC Berkeley. And for this mechanism and for many other dynamics of chemical reactions they got a Nobel Prize in 1986./nAnd Yuan Lee over here was actually by thesis advisor at Berkeley. This seems like a very peculiar kind of way for a chemical bond to form but, in fact, it is accurate. Let me talk about the energetics here of this process because that will help in trying to understand physically really what is going on in this bond formation./nBecause the first thing you're saying is, well, when that electron jumps that costs energy. We know that. We know that for a sodium atom to remove an electron here that there is an energy cost, delta E. That energy cost is what physically? The ionization energy./nThat's the ionization energy. That's 496 kilojoules per mole. But it is also true that once that electron gets in the vicinity of chlorine, the chlorine grabs it. That is the chlorine plus the electron here. When that happens some energy is released to make the chlorine minus. That energy, delta E, is minus the electron affinity./nThat was one of the definitions that we worked on a few days ago. And minus the electron affinity there is minus 349 kilojoules per mole. So you get some energy back when that electron attaches to the chlorine./nBut, overall, if I sum things up here with a sodium gas phase atom and a chlorine gas phase atom going to Na+ plus Cl-, the delta E for this reaction here overall is still positive 147 kilojoules per mole. You still have to put some energy into this system to make this reaction go. And, of course, this delta E here, I used the number 147. I added these up./nBut delta E also can be written here as the ionization of sodium minus the electron affinity of chlorine. But it's still uphill to get the electron to go from sodium to chlorine. However --/n-- the sodium and the chlorine now, once they are two ions and they come together, we're going to have some energy released because we're going to form a bond. Na+ plus Cl- going to NaCl, well, the delta E for that reaction is minus 592 kilojoules per mole./nThen overall I am going to add up this reaction to that reaction, so these are going to cancel. We have sodium gas phase plus chlorine gas phase to make sodium chloride. That is the initial reaction we were looking at, sodium and chlorine to make a sodium chloride molecule./nOverall, that delta E is minus 445 kilojoules per mole. Overall, the action is releasing energy. And that is good but things still seem a little strange, right? It still seems a little strange that this sodium is ejecting an electron and that chlorine is grabbing it and then they come together. This ejecting of an electron is a little bothersome so let's look a little more carefully at what is going on./nAn energy level diagram. Those are the energetics. And now I am going to put those energetics that I just described onto a diagram to try to make it a little bit more realistic for you. What I drew here is the energy of interaction for sodium plus a chlorine atom./nThis is the same shape that I drew for two hydrogen atoms. It's the same shape because whenever two atoms come together to form a bond, the energy of interaction looks like this. This is a general phenomenon. Way out here the two atoms are separated. The energy of interaction is zero. As they come together, there is an attractive interaction. The energy gets lower./nIt continues to get lower until we get to some value which is the bond length. It is at this point, this value of r that you have the most stable configuration. This is the chemical bond. You push them closer together. The energy goes back up. And then this well depth here, from here to here or backwards from down here to up here./nFrom down here to up there, that's the bond association energy delta E sub d. In this drawing, I reversed it. I went from here to here. It is minus delta E sub d. It's minus 445 kilojoules per mole. That's this number that I calculated right here. This is the bond strength, 445 kilojoules per mole. That's the bond strength in the sodium chloride molecule./nThat's how much energy is going to be required to take sodium chloride and pull it apart into the neutral atom sodium and the neutral atom chlorine. That is physically what that number is. But now what did I say earlier? I said that sodium ejects an electron which ends up on the chlorine./nAnd if that is the case, that is if that sodium ejects an electron that ends up on the chlorine and it does it when the sodium and the chlorine are far apart, that's going to require 147 kilojoules per mole. That is what we calculated there. That is this number. That is 147 kilocalories uphill./nSo if sodium and chlorine are really far apart, that is how much energy it is going to require to pull that electron off of sodium and put it onto the chlorine. This is IE, ionization energy minus the electron affinity. I said that that happens when it is far apart, but now the sodium ion and the chlorine ion come together. They come together to the bond length./nAnd at the bond length they form this chemical bond. And when they form this chemical bond, well, then what we have released is this much energy much 592 kilojoules per mole. Now, where did this number come from? Well, that number comes from the following simple model./nThat number comes from the potential energy of interaction between a bare plus charge and a bare minus charge at r equal 2.36 angstroms. This is the potential energy of interaction for a plus charge and a minus charge, so it's minus e squared. R here is this bond length, 2.36. If you evaluate that at r point, 2.36, you will get 592 kilojoules per mole./nSo what did I do here? I treated sodium ion and chlorine ions like two bare point charges. I forgot about the fact that sodium here has got other electrons. I forgot about the fact that chlorine here has got other electrons./nI just approximated it s2 point charges and used the Coulomb interaction between a bare plus charge and a bare minus charge to get this energy, 592 kilojoules per mole. That is where that comes from. So when the sodium and chlorine come together to this bond length, well, then they form a bond and this much energy is released within this model here./nSo we're beginning to understand what all these numbers are and physically what is going on, except maybe what is still bothering you is the fact that it still look like, in order for this reaction to go, that I've got to put in 147 kilojoules per mole into the action before I am going to get any energy back. It kind of looks like there is a barrier to this reaction from what I've told you so far./nBut the answer is there isn't because when the electron jumps is not when the sodium and the chlorine are infinitely far apart. But when the electron jumps is when the sodium and chlorine are right here at this value of r star. Why right here?/nWell, it happens right here because of this. This blue line here, see this blue line? What that is the Coulomb energy of interaction as a function of r between two point charges, plus one and minus one. So way out here when r is very large that energy of interaction for these two ions is zero./nBut as these two ions come closer together that energy of interaction becomes more and more negative. There is a one over r dependence here. And that is what that blue line approximates. At some point that Coulomb interaction energy crosses this black curve here which represents the interaction energy between a neutral atom and a chlorine atom./nRight here the Coulomb interaction energy is the energy difference between up here to down here. Right here this is the Coulomb interaction energy minus E squared four pi epsilon knot at r star now, not equilibrium distance./nAt r star where the electron jumps. Right here, this Coulomb energy of interaction equals this energy difference between the two separated ions and the two separated atoms. This energy difference here measured from here to here is minus the ionization energy minus the electron affinity. So right here this electron can jump and it doesn't cost us any energy./nBecause the Coulomb interaction has already set in. Right here that Coulomb interaction energy is equal to this energy difference. That is when the electron jumps, right here. And then it is at that point that the sodium and the chlorine are accelerated together up to the equilibrium bond length form of bond and you have the sodium chloride molecule./nLet's calculate what this value of r star is. At what value of r does this electron actually jump? Well, we can do that. This is minus e squared over 4 pi epsilon knot r star. That is going to be equal to minus the quantity ionization energy of sodium minus the electron affinity of chlorine./nLet me rearrange this here. I am going to solve for r star. R star is equal to e squared over four pi epsilon knot times the quantity ionization energy of sodium minus the electron affinity of chlorine./nR star is equal to e squared. E squared is 1.602 times ten to the minus nineteen Coulombs squared over four pi epsilon knot, you can look up epsilon knot, times this difference in energy./nThe ionization energy of sodium minus the electron affinity of chlorine, which way over here we said was 147 kilojoules per mole. So let me put that into joules so my units are consistent, 1.47 times ten to the fifth joules per mole. But now be careful here. When you calculate our star, this is for a molecule./nNot for a mole of molecules. I've got to change all of these things that are in here per mole to molecules, so I have got to multiply this by 6.022 times ten to the twenty-third molecules per mole. And then the value of r star is equal to 9.45 times ten to the minus ten meters./nThat is when the electron jumps. Let's draw a picture of what actually the relative size is here. So we have a sodium atom. That sodium atom has a diameter of about 3.8 angstroms. And we have a chlorine atom. That chlorine atom has a diameter of about two angstroms./nIt is 9.45 angstroms. That is the distance between those two neutral species, when that electron jump process actually happens. So they are pretty far apart, pretty far apart compared to the bond length of 2.36 angstroms./nAnd, indeed, it turns out that this number 9.45 angstroms is very, very close to what is measured experimentally when that electron jump process happens. This simple model treating sodium and chlorine as point charges works, but it works because sodium chloride is a very ionic bond. This simple model will not work for bonds that are not very ionic./nAll right? OK. See you Friday.
Channels: Chemistry (General)
Uploaded by: mitlectures ( Send Message ) on 16-04-2009.
Duration: 50m 10s